

Rearrangements and combinations of these equations
yield a set of relationships which describe motion whenever the acceleration
is constant. Two of these relationships called the equations of motion allow
the calculation of speed and position at any time if the initial position
and speed are known.
Whenever the speed change is steady, the acceleration
is constant. There are many physical situations when acceleration is constant;
an object falling in gravity, or pushed by a constant force. A constant
force produces a constant acceleration (steady speed change). This relationship
between force and motion has been formalized in Newton's Second Law.
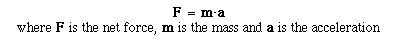
Newton's law in this form is used to define force, but the law is perhaps
more intuitive when written it terms of the acceleration.

When an unbalanced force acts on an object,
the force does work and the object changes its speed. The work done by the
net force depends simply on the force and the distance moved.
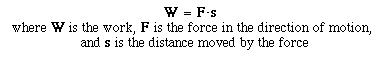
The work done by the net force changes the kinetic energy of an object.
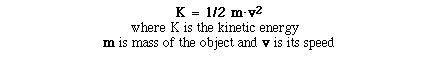
Energy is often stored and moved around electrically. When energy carried
by electricity, that energy is not measured directly, but may be determined
from measurements of current, voltage, and time.


Graphs of speed vs. time supplement and clarify the mathematical model
of motion. For these graphs, most everybody is in the habit of using the
horizontal axis for time. Mathematicians and scientists are in the habit
of using the horizontal axis for the independent variable. These customs
are in conflict when time is allowed to be dependent variable. We choose
to plot time on the horizontal axis in keeping with ordinary habit, partly
because this conveniently makes the slope of a time graph equal a rate of
change. Time graphs are continuous functions which show how change plays
out as time flows forward. The vertical location of a point on a time graph
tells the size at that time, and the slope of a time graph tells the rate
of change.

On a graph of s vs time, the slope tells the rate at which s
changes with time. If s represents position, then the slope is the
speed.